Solutions to Irodov problems

Problems In General Physics, I.E. Irodov, Part-I Physical Fundamentals in Mechanics

Chapter 1.1 Kinematics

10 interesting problems in elementary mechanics

This post intends to provide 40 interesting problems in elementary mechanics from IE Irodov, Problems in general physics that I solved in last couple weeks.

Note that another 30 problems — additional 12 at hand, which has been solved will be uploaded after they are scanned, shortly.

Irodov cover 2018-10-01 — Go to Amazon to view the book. Click on Left.

Basic Concepts

In this class today we will discuss the subject of “kinematics” briefly and solve some problems, based on the same.

The motion of objects are studied under the heading “mechanics“.

a. Mechanics is called “kinematics” if we study about the “nature of motion” without regard to what factors are causing such motion.

b. In addition to kinematics, when we focus our attention to study the factors that cause motion, such is named as dynamics.

In kinematics today we will discuss a few problems that will cover the topics of

A. Relative velocity — $\boxed{\Delta \vec{v} = \vec{v}_{21} = \vec{v}_2 - \vec{v}_1}$

B. Average velocity — $\boxed{\bar {\vec{v}} = \frac{\vec{v}_2 - \vec{v}_1}{t_2 - t_1}}$

C. Motion under uniform acceleration — $\boxed{\vec{a} = 0}$ , Equations of motion — $\boxed{\vec{v} = \vec{u} + \vec{a} \,t \,etc }$

D. Position and velocity as vector — $\boxed{\vec{v},\, \vec{r}}$

E. Resolving a vector into components — $\boxed{\vec{R} = \vec{A} + \vec{B}}$

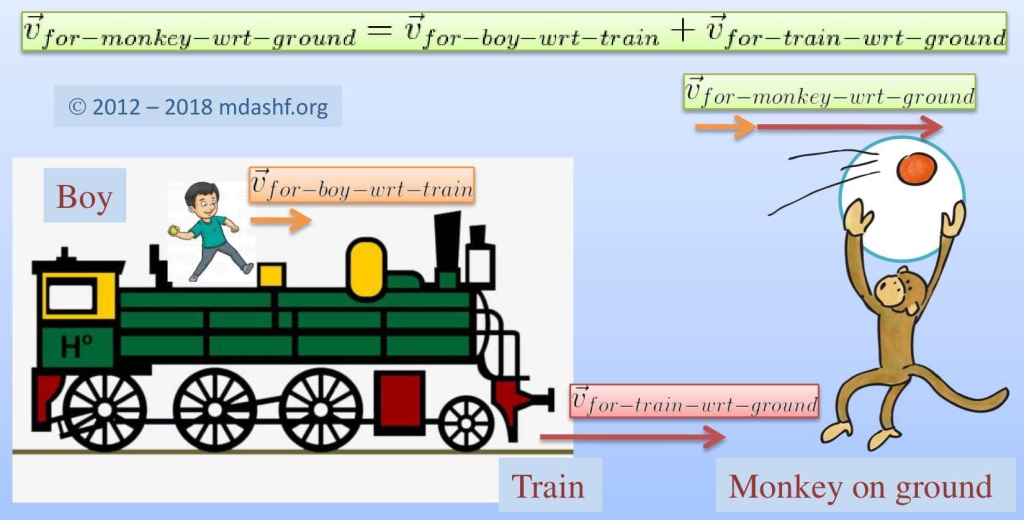
A. Relative velocity

Lets begin by briefly discussing the case of relative velocity of two objects which are either

a. moving along in one direction at given velocities, or

b. are moving in opposite directions, making an angle of $180^0$ with each other.

Lets say object A is moving with velocity $\vec{v}_A$ and object B is moving along the same direction as A with velocity $\vec{v}_B$ .

We would consider only the scalar magnitudes of these vectors $\vec{v}_A$ and $\vec{v}_B$ ; ${v}_A$ and ${v}_B$ .

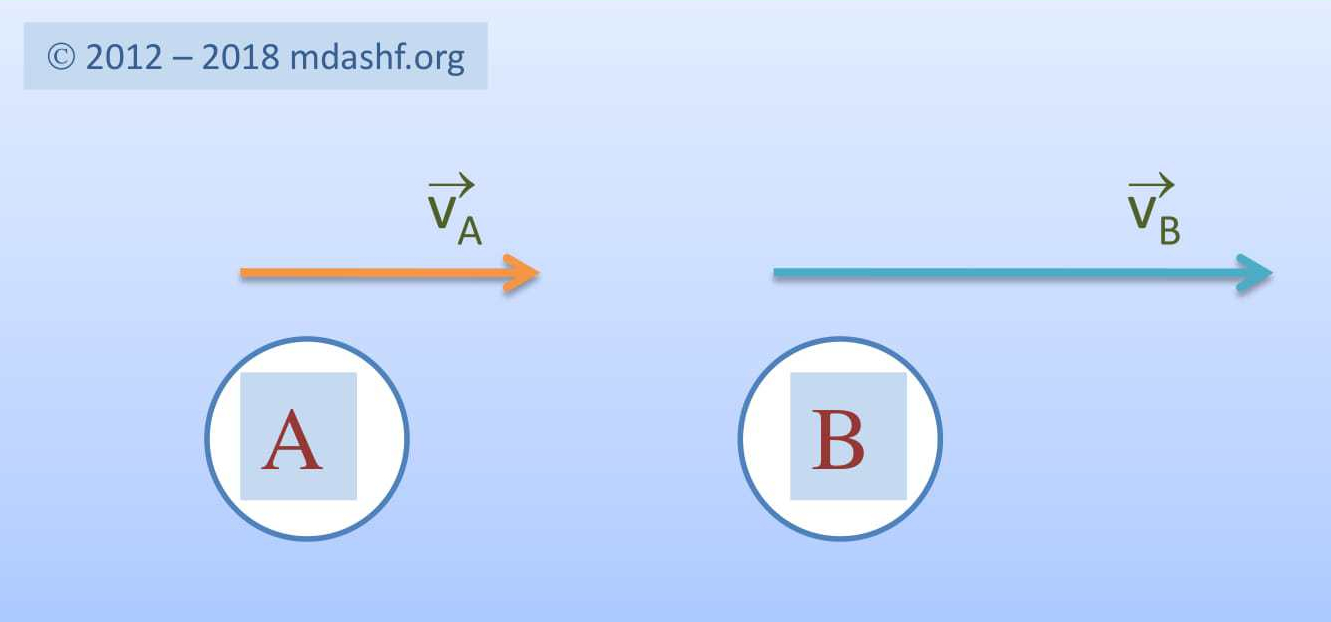
vectors1_edited

As seen here $\vec{v}_A$ is the velocity of A wrt a specified frame-of-reference; here ground. Similarly B is moving wrt a specified f-o-r; here ground, at velocity $\vec{v}_B$ .

We like to know at what velocity B is moving with respect to a frame fixed onto A — which is moving at $\vec{v}_A$ , or vice-a-versa.

Its easy to see that wrt B, A is moving at a speed given by $\vec{v}_{BA}$ where; $\vec{v}_{BA}=\vec{v}_B - \vec{v}_A$ . So in our simple case of parallel motion of A and B; $v_{BA}=v_B - v_A$ .

Train and ball example

If a train is moving at a speed of $50\frac{m}{s}$ wrt platform and in the train a ball is thrown along the motion of the train at a speed of $20\frac{m}{s}$ wrt ground — here platform, we can see that:

$\boxed{v_{ball-wrt-train}=v_{ball-wrt-ground} - v_{train-wrt-ground}=20\frac{m}{s}-50\frac{m}{s} = -30\frac{m}{s}}$

In other words the ball has been thrown at a speed of $30\frac{m}{s}$ opposite to trains direction (of motion) and not along it as reported by someone on the ground.

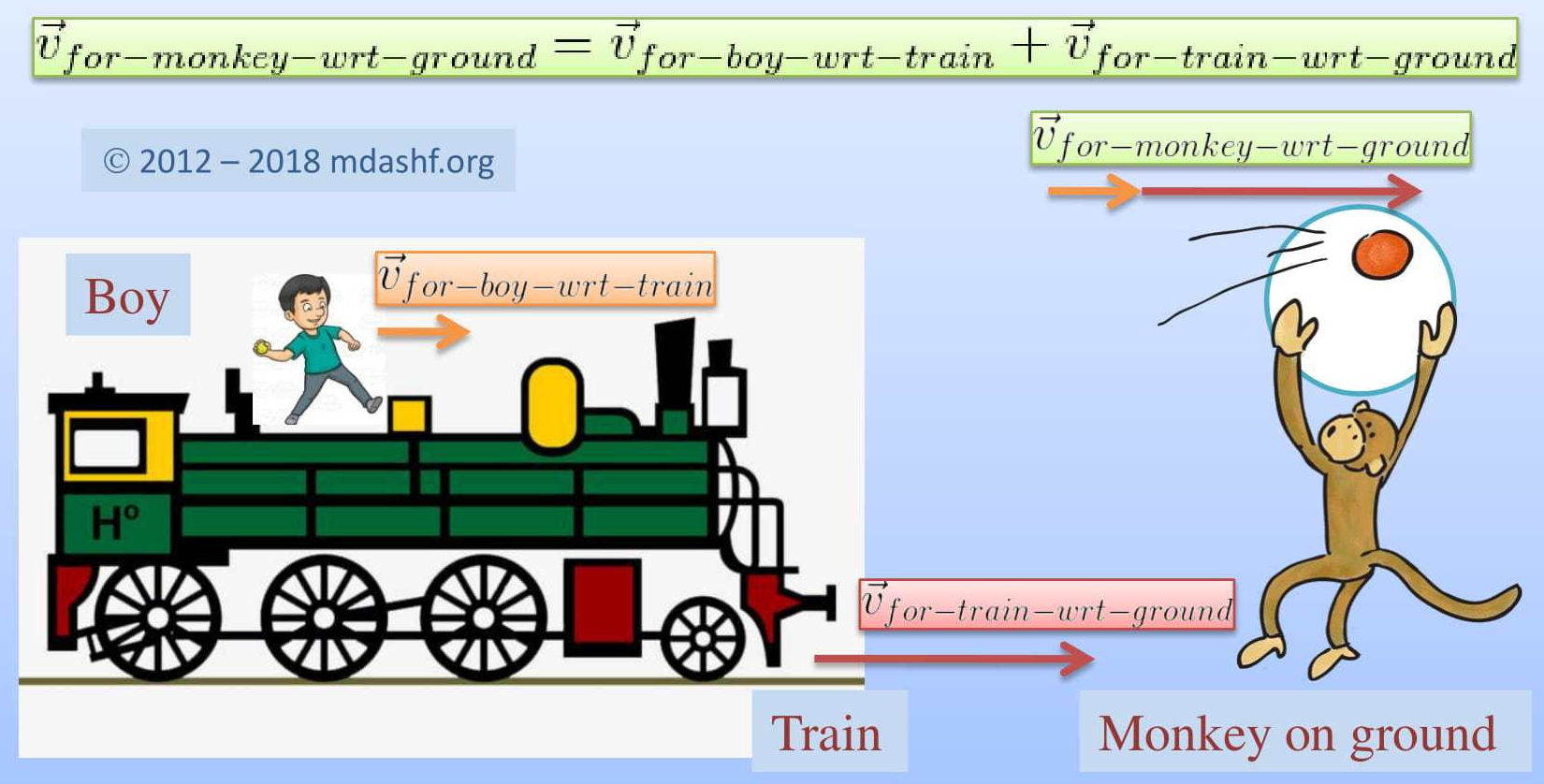
a. We say the motion is relative.

b. Such addition of velocities are known as Galilean relativity, velocity addition — of Newtonian or Galilean mechanics, or simply relative velocity.

c. All vectors, not just velocity vectors add up under such conditions of relative motion.

d. If the ball were to be thrown along the direction of the train’s motion at lets say $20\frac{m}{s}$ then someone on the ground would observe the speed of the motion to be $(50+20)\frac{m}{s}=70 \frac{m}{s}$ .

Now lets solve a real life problem.

Problem 1 — Irodov 1.1

A motorboat going downstream overcomes a raft at a point A; $\tau = 60\, minute$ later it turned back and after sometime passed the raft at a distance $l = 6.0 \, km$ from the point A.

Find the flow velocity assuming the duty of the engine to be constant.

Answer to Problem 1

Lets first understand the problem.

The raft is something which is assisted by the flow-velocity of the water;

so, $v_w = v_r = v$ .

The motor boat can have its own speed $v_b$ . But along the stream it will have a relative velocity — wrt ground, given by “velocity addition rule“.

a. Downstream: $v_b +v$ .

b. Upstream: $v_b -v$

The raft has traveled $6 \, km$ from A, by the time motor boat has met it on its way back; $\boxed{v(1+ \tau) = 6 \, km \hspace{15pt}eq^n \,(1)}$ .

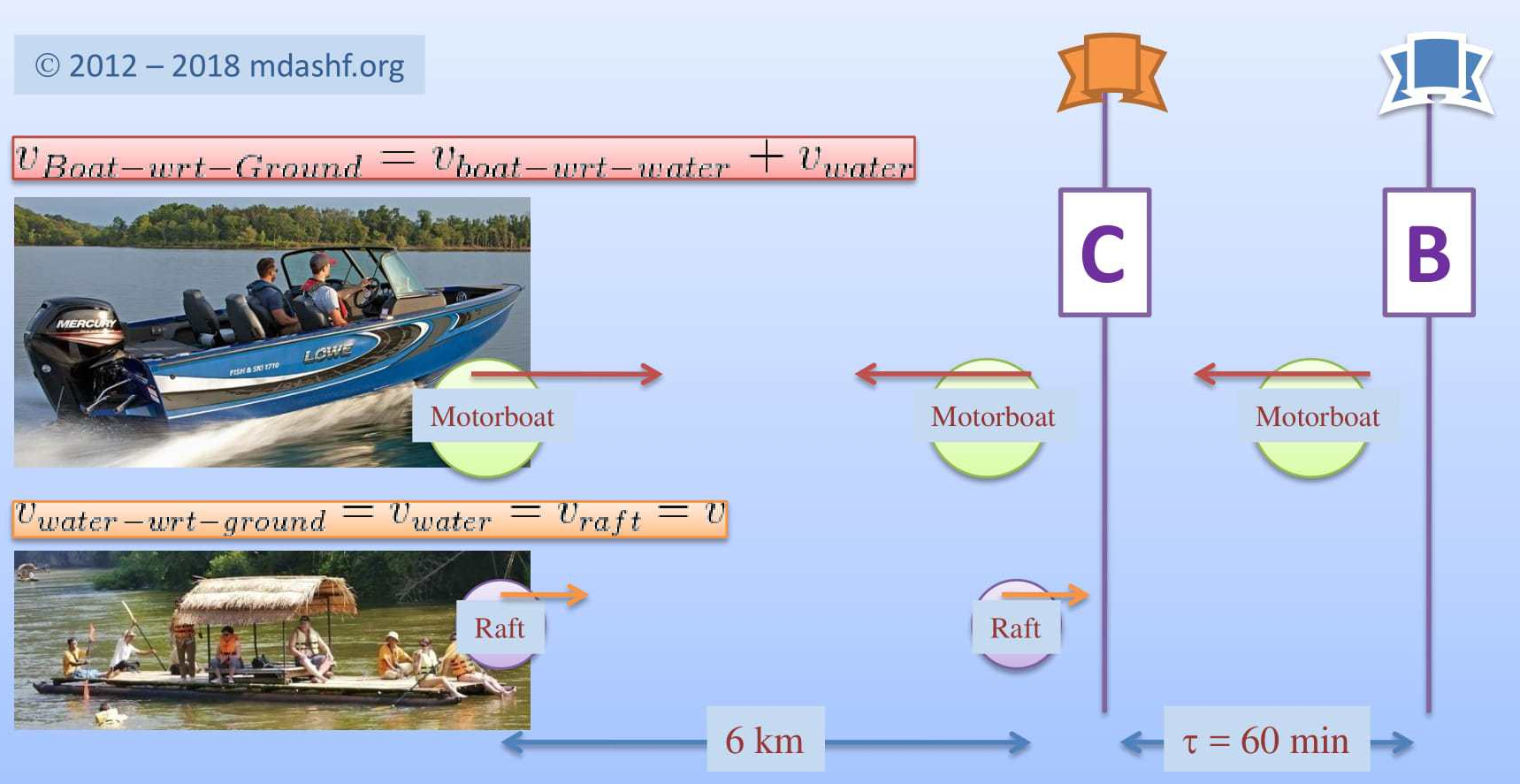
boat_relative_edited — The diagram has a slight hitch, will be fixed anon. The time $\tau$ should be shown from starting point (A, unmarked) to B. Not from C to B.

The diagram has a slight hitch, will be fixed anon. The time $\tau$ should be shown from starting point (A, unmarked) to B. Not from C to B.

So $\boxed{(v+v_b).1 - (v-v_b).\tau = 6 \,km\hspace{15pt}eq^n\,(2) }$ .

From $eq^n \,(1) \, \&\, eq^n \,(2)$ , $(v+v_b).1 - (v_b-v).\tau = v(1+\tau)$ or $v(1+\tau) + v_b(1-\tau) = v(1+\tau)$ .

⇒ $v_b(1-\tau) = 0$ , or; $\tau = 1 \, hr$ as $v_b \ne 0$ .

So $v(1+1) = 6 \,km$ , from $eq^n \,(1)$ , this gives $v = 3 \frac{km}{hr}$ .

B. Average velocity

Now let us consider the idea of average velocity and average acceleration.

a. We define average velocity as the total displacement divided by total time taken. Its a vector quantity which is not accurate if motion is non-uniform.

$\boxed{<\vec{v}>=\frac{\Delta \vec{r}}{\Delta t}}$ .

b. For uniform motion, in 1-dimension, average velocity gives a scalar magnitude which is called as average speed.

c. Displacement is a vector which is the difference of two position vectors.

$\boxed{\Delta\vec{r}=\vec{r_2}-\vec{r_1}}$ .

d. Distance is the scalar magnitude of displacement if we consider uniform speed along 1-dimension: $\Delta r = |\Delta\vec{r}|=s=x$ .

e. By uniform we mean covering of an equal distance in an equal interval of time. This means speed is constant.

So average speed is total distance covered divided by total time taken for covering the distance.

$\boxed{= \frac{\Delta r}{\Delta t} = \frac{s}{t}}$ .

Average acceleration

In the same way we define “average acceleration“.

Acceleration is a vector given by the total change in velocity vector in a given interval of time.

Thus

$\boxed{<\vec{a}>=\frac{\Delta \vec{v}}{\Delta t}}$ and $\boxed{=\frac{\Delta v}{\Delta t}=\frac{v}{t}}$

C. Motion under uniform acceleration — equations of motion

a. For a motion where, acceleration is constant or uniform, we can derive a set of relation called as “kinematic relations” or “equations of motion“.

b. Lets do so in the 1-dimensional uniform acceleration case. We only state the results here, without derivation.

$\boxed{v=u+at \,\,\,or\,\,\,v=v_0 + at}$ .

$\boxed{s=ut+\frac{1}{2}at^2 \,\,\,or\,\,\,x=x_0 + v_0 t+ \frac{1}{2}at^2}$ .

$\boxed{v^2 = u^2 +2as \,\,\,or\,\,\,v^2 = v_0^2 +2as}$ .

Now lets solve two problems based on the above.

Problem 2 — Irodov 1.2

A point traversed half the distance with a velocity $v_0$ . The remaining part of the distance was covered with velocity $v_1$ for half the time, and with velocity $v_2$ for the other half of time.

Find the mean velocity of the point averaged over the whole time of motion.

Answer to problem 1.2

average_vel_edited

In the first leg of the journey, point moves from A to B, at speed $v_0$ and covers distance $\frac{x}{2}$ . If it takes time $t'$ , then; $t'\times v_0 = \frac{x}{2}$ — this we infer from the definition of average velocity.

So $\boxed{t'= \frac{x}{2v_0}\hspace{15pt} eq^n\,(1)}$ .

For second leg of the journey, i.e., B to C and C to D; $\frac{t}{2} \times v_1 + \frac{t}{2} \times v_2 = \frac{x}{2}$ .

or $\boxed{t = \frac{x}{v_1 + v_2}\hspace{15pt} eq^n\,(2)}$ .

From $eq^n\, (1) \,\,\&\,\,eq^n\,(2)$ , $t' + t =x[ \frac{1}{2v_0}+\frac{1}{v_1+v_2}]$ .

or $\frac{x}{t' + t} = \frac{(v_1+v_2)v_0\times 2}{2v_0 + (v_1+v_2)}$ .

so $\boxed{ = \frac{2v_0(v_1+v_2)}{2v_0 + (v_1+v_2)}}$ .

Problem 3 — Irodov 1.3

A car starts moving rectilinearly, first with acceleration $a = 5.0 \,\frac{m}{s^2}$ — with initial velocity zero, then uniformly and finally decelerating at the same rate $a$ , comes to a stop.

The total time of motion equals $\tau = 25 \,$ . The average velocity during that time is equal to $= 72 \, \frac{km}{h}$ . How long does the car move uniformly?

Answer to problem 1.3

We should get a final answer: $\boxed{t_2^2 = \tau^2 [1-\frac{4}{a\tau}], \, t_2 = 15s}$ .

Lets see how.

Lets implement the kinematic relations for the three legs of the journey represented by the times $t_1, t_2, t_3$ .

Leg 1: $s_1 = \frac{1}{2} at_1^2, \,v_1=0,\,v_2=at_1$ .

Leg 2: $s_2 = v_2t_2=at_1t_2, \, as \, v_2=at_1$ .

Leg 3: $s_3 = \frac{1}{2}at_3^2, \,v_3=0$ .

Now let us apply another kinematic relation, for the 3rd phase — or leg of the journey, represented by time $t_3$ .

$v^2 = u^2 - 2as \,\, or \,\,v_3^2 = 0 = v_2^2 - 2as_3$ .

⇒ $(at_1)^2 = 2as_3 \,\, \,\, t_1^2 = \frac{2s_3}{a} = t_3 ^2$ as $t_1 = t_3$

Now $= \frac{s_1 + s_2 +s_3}{t_1+t_2+t_3}=\frac{s}{\tau}$ with $\tau=25=t_1+t_2+t_3$ so, $= \frac{\frac{1}{2}at_1^2 + at_1t_2 + \frac{1}{2}at_3^2}{\tau}$ .

So, $= \frac{\frac{1}{2}at_1^2 + at_1t_2 + \frac{1}{2}at_1^2}{\tau}$ as $t_1 = t_3$ so, $= \frac{at_1^2 + at_1t_2}{\tau}=\frac{at_1(t_1+t_2)}{\tau} = \frac{at_1(\tau-t_3)}{\tau} \,\, as \,\, \tau = t_1+t_2+t_3$ .

$t_2 = \tau - (t_1+t_3) = \tau - 2t_1, \,\, \,\, t_1 = \frac{\tau -t_2}{2}, \,\tau-t_3 = \tau - t_1 = \frac{25+t_2}{2}$

$= \frac{a}{\tau}\big( \frac{\tau - t_2}{2}\big)\big( \frac{\tau + t_2}{2}\big)=\frac{a}{4\tau} (\tau^2 - t_2^2)$ so $\frac{4\tau }{a} = \tau^2 -t_2^2$ .

Thus, $t_2^2 = \tau^2 - \frac{4\pi \tau^2 }{a\tau}=\tau^2 \big ( 1-\frac {4\pi }{a\tau} \big)$ as we claimed in the beginning.

We have $a = 5 \frac{m}{s^2}, \,\, \tau = 25 s, \,\, = 72 \frac{km}{hr}= \frac{72\times 10^3}{3600}\frac{m}{s}$ .

This gives $t_2 = 15\,s$ us for time during which there was uniform motion.

D. Position and velocity as vectors

Motion of objects are described by vectors, we defined position vector $\vec{r}$ and velocity vector $\boxed{\vec{v}=\frac{\vec{r}}{t}\,\,or \,\, \frac{\Delta \vec{r}}{\Delta t}}$ .

Lets illustrate this by a problem.

Problem 4 — Irodov 1.5

Two particles 1 and 2 move with constant velocities $\vec{v_1}$ and $\vec{v_2}$ . At the initial moment their radius vectors are equal to $\vec{r_1}$ and $\vec{r_2}$ .

How are these 4 vectors to be related for the particles to collide.

Answer to Problem 4 — Irodov 1.5

For two objects to collide their relative velocity must occur in the direction of their relative displacement. The vectors make it into a one-dimension like condition, where collision occurs if the objects move towards each other in the same direction.

Lets cast the problem into the following diagram.

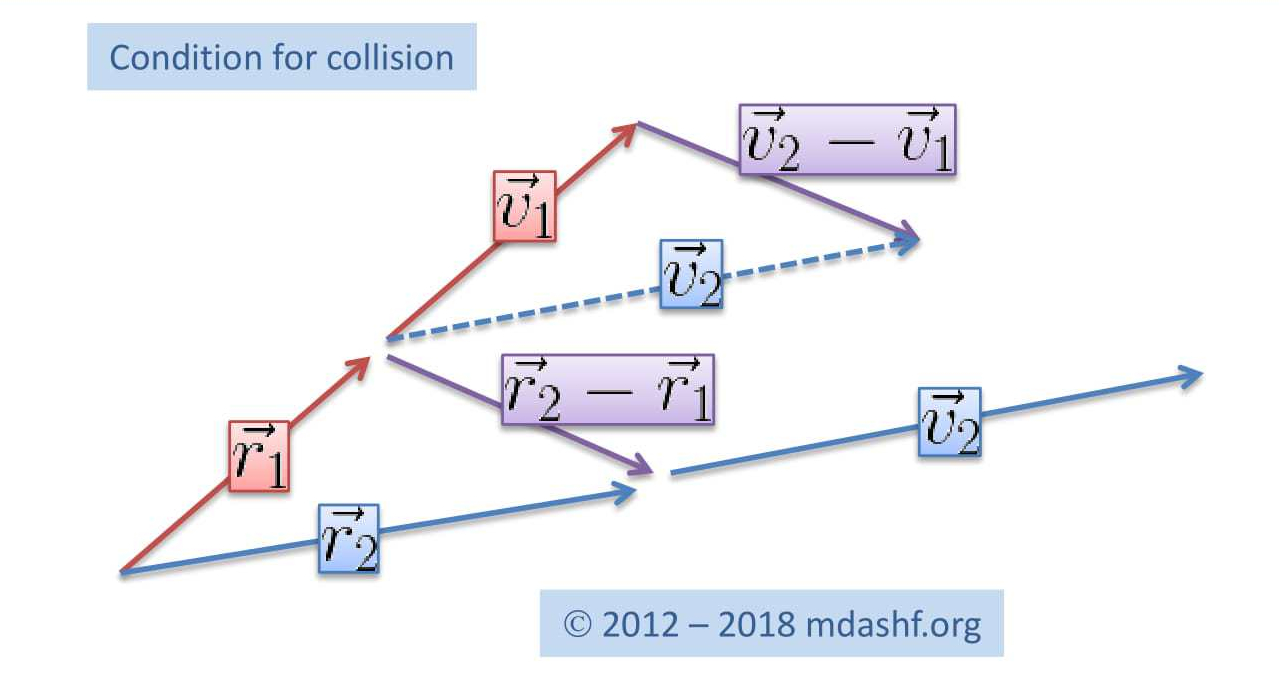
Evidently the condition of collision is satisfied when: $\boxed{\frac{\Delta \vec{v}}{|\Delta \vec{v}|}=\frac{\Delta \vec{r}}{|\Delta \vec{r}|}}$

which is a shorthand for $\boxed{\frac{\vec{v_2}-\vec{v_1}}{|\vec{v_2}-\vec{v_1}|}=\frac{\vec{r_2}-\vec{r_1}}{|\vec{r_2}-\vec{r_1}|}}$ .

E. Resolving a vector into its components

Physical quantities can be expressed as vectors. Vectors are important mathematical tools. We state couple results that relate two vectors — components, to their resultant vector.

Cosine law gives magnitude of a resultant vector from its components, by using the dot product of the resultant vector with itself as the square of its magnitude.

A. Law of cosines

The magnitude of resultant of two vectors is given as follows.

$\boxed{R = \sqrt{A^2 + B^2 + 2AB\cos\theta}; \,\,\, for \, \vec{R} = \vec{A} + \vec {B} , \angle (\vec{A}, \,\vec{B}) = \theta }$ .

B. Law of sines

The angle made by resultant R with component A is given by $\boxed{\sin\alpha = \frac {B}{R} \sin \theta }$ and $\boxed{\tan\alpha = \frac {B}{A+B\cos\theta} \sin \theta }$ .

Lets employ this important result to see how useful it is towards “everyday” life.

Problem 5 — Irodov 1.6

A ship moves along the equator to the east with velocity $v_0 = 30\, \frac{km}{h}$ . The south-eastern wind blows at an angle $\phi = 60^0$ to the equator with velocity $v=15 \,\frac{km}{h}$ .

Find the wind velocity $v'$ relative to the ship and the angle $\phi '$ between the equator and the wind direction in the reference frame fixed to the ship.

Answer to problem 5 — Irodov 1.6

$\vec{v'}=\vec{v}+\vec{v_0}$ , so, $v' = \sqrt{v^2 + v_0^2 + 2vv_0 \cos \phi}=40 \, \frac{km}{h}$

$\sin\alpha = \frac{v_0}{v}\sin\phi=\frac{30}{40}\sin 60^0$

$\alpha = \sin^{-1}(\frac{3\sqrt{3}}{8})= \sim 41^0$

$\boxed{\phi ' = 60^0 - \alpha = 60^0 - 41^0 = 19^0}$ .

Problem 6 — Irodov 1.7

Two swimmers leave point A on one bank of the river to reach point B lying right across on the other bank. One of them crosses the river along the straight line AB while the other swims at right angles to the stream and then walks the distance, that he has been carried away by the stream to get to point B.

What was the velocity $u$ of his walking if both swimmers reached the destination simultaneously? The stream velocity $v_0 = 2.0 \, \frac{km}{hour}$ and the velocity $v'$ of each swimmer with respect to water equals $2.5\, \frac{km}{hour}$ .

Answer to problem 6 — Irodov 1.7

Notes

A. Answer stated in Irodov is $\boxed{u = \frac{v_0}{\sqrt{1-({v_0^2}/{v'^2})}-\,1} = 3.0 \, \frac{km}{hour}}$ .

B. Derivation is given in solution manual from A.K. Singh

C. We will derive in another fashion — without looking into A.K. Singh, this you can call method by M. Dash, the author of the present discourse.

D. If we recast $\cos \theta$ etc in M. Dash method into $v_0, v'$ etc we will get A.K. Singh method, as well as Irodov’s final formula.

E. Understand carefully the concept of relative velocity — eg when taking ratio of sides in the diagram, do not use values from two different frames of references. We will use “wrt ground frame” eg.

Lets begin with a diagram, I will explain the parameters and the details of the steps.

Here is the required diagram.

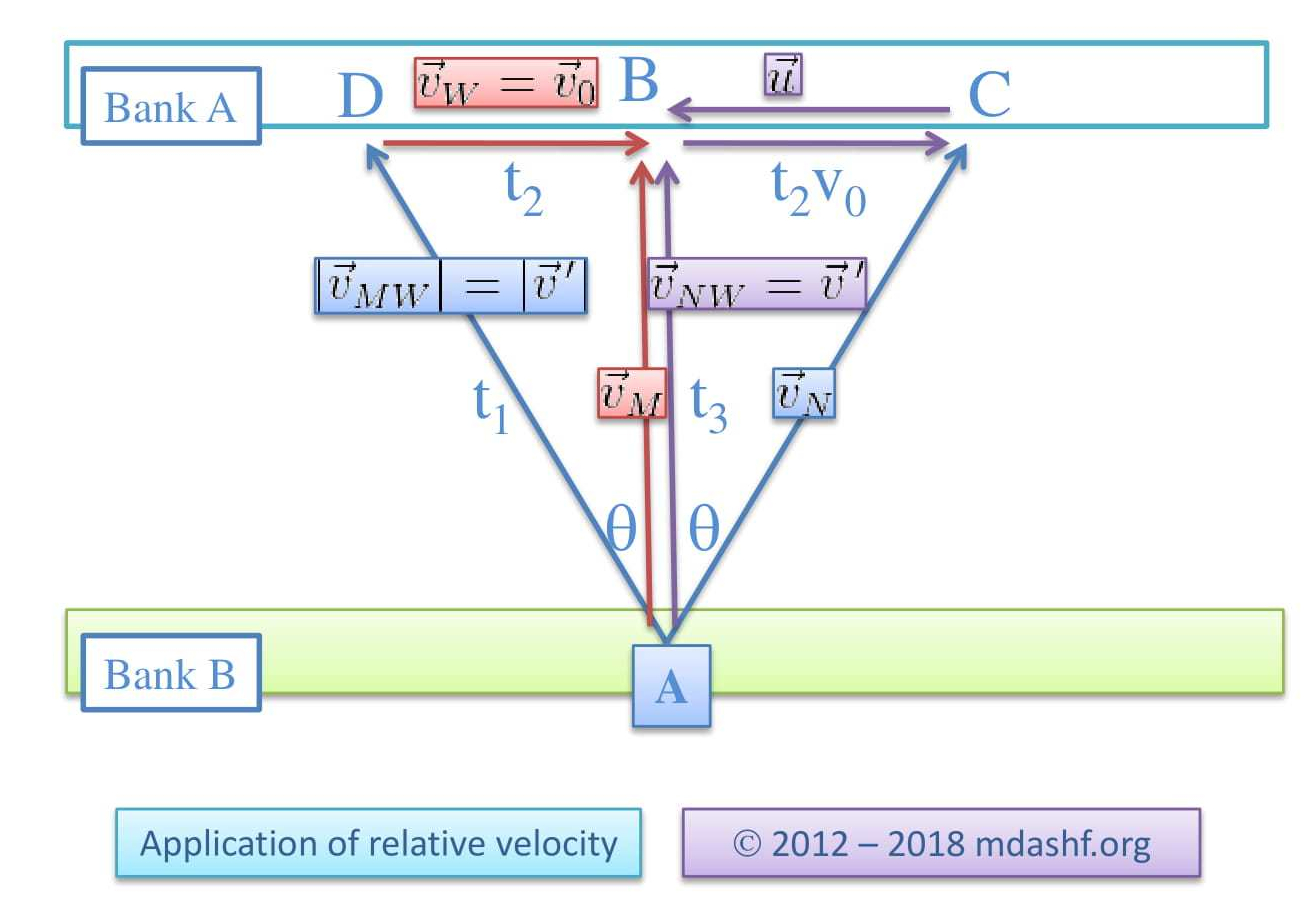
relative_velocity_problem_edited — Take help from the color coding to avoid misunderstanding.

Person M goes along AD, drifts to B simultaneously — due to ${\vec{v}}_W = {\vec{v}}_0$ . His velocity wrt ground is ${\vec{v}}_M$ which is a vector sum of his velocity wrt water — ${\vec{v}}_{MW}={\vec{v}}^{\,'}$ and of velocity of water wrt ground — ${\vec{v}}_W={\vec{v}}_0$ .

$\vec{v}\,'$ and $\vec{v}_0$ are Irodov’s notation. In our notation “MW” stands for “of M wrt W=water”. We drop index for “wrt ground = G“.

So ${\vec{v}}_M$ is “of M wrt ground”. Similarly for person N, wrt water: ${\vec{v}}_{NW}={\vec{v}}^{\,'}$ — except directional adjustment, magnitude is same, it would be inconsistent to define two different vectors with one symbol and ${\vec{v}}_N$ is wrt ground.

Concept of relative velocity: resultant vectors of “velocities wrt water” and velocity of water wrt ground.

$\boxed{\vec{v}_M=\vec{v}_{MW}+\vec{v}_W \hspace{15pt} eq^n\,(1) \,\, \,\, \vec{v}_N=\vec{v}_{NW}+\vec{v}_W \hspace{15pt} eq^n\,(2)}$ .

Person M would take time $t_1$ for path AD — wrt water, and time $t_2$ for path DB — wrt ground, due to water velocity. These times don’t add up, because they are variables in two different frames.

Person N would similarly take time $t_3$ for path AB — wrt water, and time $t_2$ for path BC — wrt ground. The excess time between $t_1$ and $t_3$ — because $AD > AB$ , is to the advantage of N.

This excess time is used by N to cover distance CB so that M and N reach point B simultaneously. — Time $t_1$ is cancelled out and we need not bother about it.

Now let us solve the problem from this much information.

$\boxed{2.5 t_1 = AD \hspace{15pt} eq^n\,(3) \,\, \,\, 2.5 t_3 = AB \hspace{15pt} eq^n\,(4)}$ .

Note that $t_3$ is the time during which N would cover the distance AB given to its speed of $v\,'=2.5\, km/hr$ — this matches with $eq^n \,(4)$ .

Also ground speed of M is $\frac{AB}{t_1}$ . But $AB = \sqrt{{AD}^2 - {DB}^2}$ . AD is covered at speed $v'=2.5 \,\frac{km}{hr}$ in time $t_1$ . Also DB is covered in time $t_1$ at speed of flow of water = $v_0 = 2.0 \,\frac{km}{hr}$ .

Thus we have two equations.

$\frac{AB}{t_1}=\frac{3}{2}, \,\, \frac{AB}{t_3} = \frac{5}{2}$ .

Thus we get $\cos\theta = \frac{AB}{AC}=\frac{AB}{BD}=\frac{t_3}{t_1}=\frac{3}{5}\hspace{15pt}eq^n\,(5)$ .

From $eq^ns \,3,\, 4 \, \&\, 5$ we get: $\boxed{2.5(t_1-t_3)=|AC-AB| =|AB(1-\frac{1}{\cos\theta})| \hspace{15pt}eq^n\,(6)}$ .

$u$ = speed of N, wrt ground, covered in time $t_1-t_3$ is $\frac{BC}{t_1 - t_3}$ .

From $eq^n\,(6)$ we have $2.5 = \frac{AB}{t_1-t_3}\big( \frac{\cos\theta -1 }{\cos\theta}\big)$ .

or $\frac{2.5\cos\theta}{\cos\theta -1} = \frac{AB}{BC}\frac{BC}{t_1-t_3}$ .

So, $\frac{BC}{t_1-t_3} = \frac{AB}{BC}\big[ \frac{2.5\cos\theta}{\cos\theta -1} \big] \hspace{15pt} eq^n\,(7)$ .

From $eq^n\,(7)$ we have $u=\frac{BC}{t_1-t_3} = \frac{2}{2.5}\big[ \frac{2.5\times 3/5}{3/5 -1} \big]=3.0 \,\frac{km}{hour}$ .

Note that AB is covered at speed wrt water. BC is covered at speed of water wrt ground.

That’s the answer.

Problem 7 — Irodov 1.8

Two boats, A and B, move away from a buoy anchored at the middle of a river along the mutually perpendicular straight lines: the boat A along the river, and the boat B across the river. Having moved off an equal distance from the buoy the boats returned.

Find the ratio of time of motion of boats $\frac{\tau_A}{\tau_B}$ if the velocity of each boat with respect to water is $n = 1.2$ times greater than the stream velocity.

Answer to problem 7 — Irodov 1.8

G: frame of reference at rest wrt ground. W: frame of reference associated with speed of water.

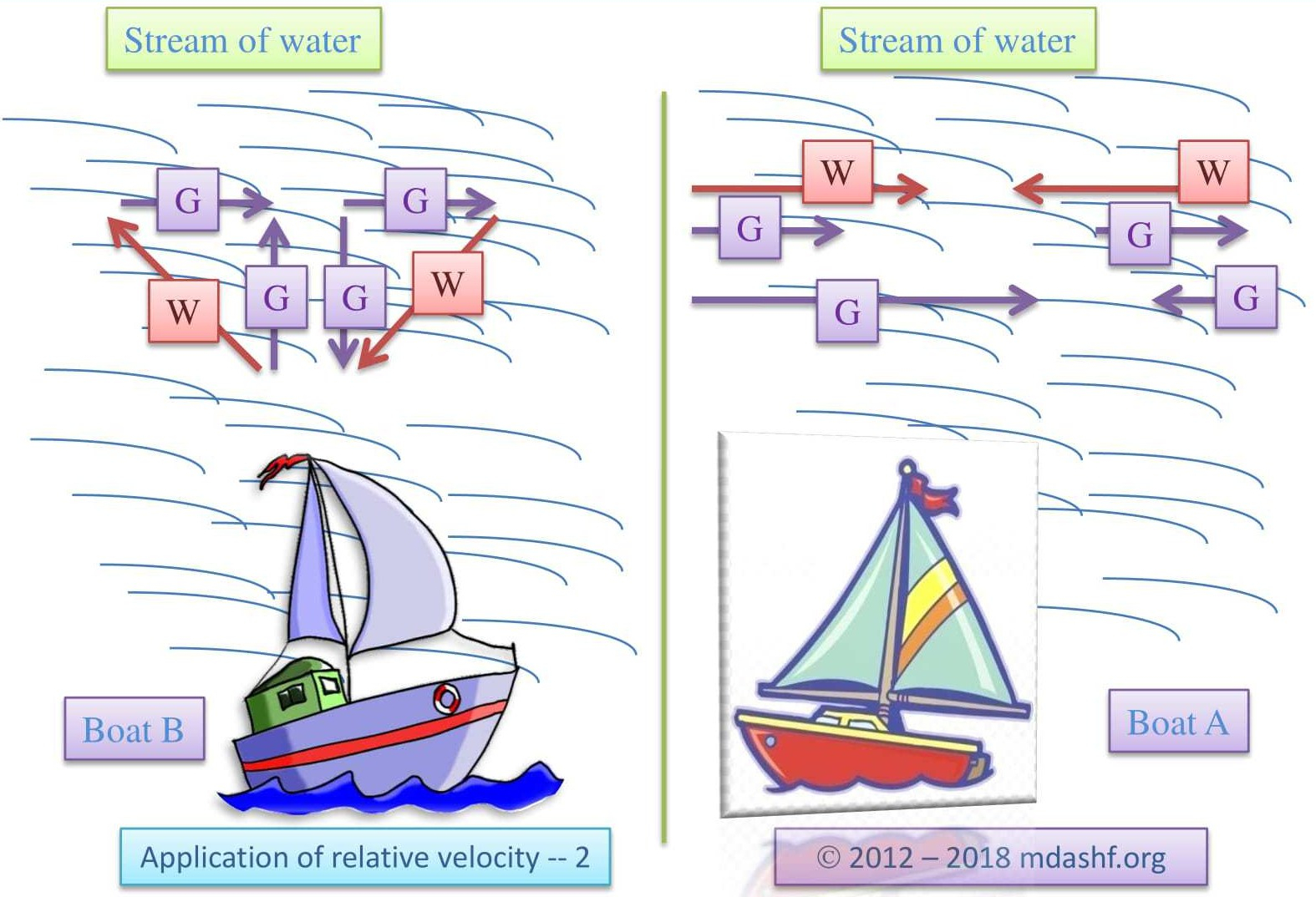
The situation is described above. For boat A, it goes assisted by stream velocity and comes opposed by it, both for distance $x$ .

Boat B on the other hand goes at an angle with the stream velocity so drift of the stream brings the motion perpendicular — $\perp$ , to the stream velocity, straight up.

It’s reverse motion has the same resultant or relative velocity wrt the ground.

Note that G represents wrt ground based frame and W represents wrt water-flow based frame.

Now let us solve the problem: $\tau_A =\frac{x}{v_A} + \frac{x}{v_A'}=x\Big(\frac{1}{v_A} + \frac{1}{v_A'}\Big)$ .

$v_A, \, v_A\,'$ are relative velocity wrt ground frame.

$v_A =(n+1)v_W, \, v_A'=(n-1)v_W$ where $v_W$ is stream velocity which is a ground-frame based vector.

So $\tau_A = \frac{x}{v_W} \big( \frac{n+1+n-1}{n^2-1} \big) =\frac{2xn}{v_W (n^2-1)}$

For B, $\tau_B=\frac{x}{v_B}+\frac{x}{v_B}=\frac{2x}{v_B}$ .

$v_B=\sqrt{v_{BW}^2-v_W^2}=\sqrt{(n^2-1)}v_W$ .

So, $\frac{\tau_A}{\tau_B}=\frac{2xn}{v_W(n^2-1)}\times\frac{\sqrt{n^2-1}\, v_W}{2x}=\frac{n}{\sqrt{n^2-1}}=1.8$ .

Boat A is late wrt Boat B for the travel of same distance $2x$ .

Problem 8 — Irodov 1.9

A boat moves relative to water with a velocity which is $n=2.0$ times less than the river flow velocity.

At what angle to the stream direction must the boat move to minimize drifting?

Answer to problem 8 — Irodov 1.9

a. Our answer is: $\boxed{\theta = \frac{\pi}{2}+\sin^{-1}(\frac{1}{n})}$ .

b. I derive this answer in a totally different method from what A.K.Singh has worked out. Singh uses minimization rule i.e. he sets derivative to zero. I use the direct relative velocity rule — i.e. law of sines for relative velocity, check a few pages above.

Lets begin by the diagram.

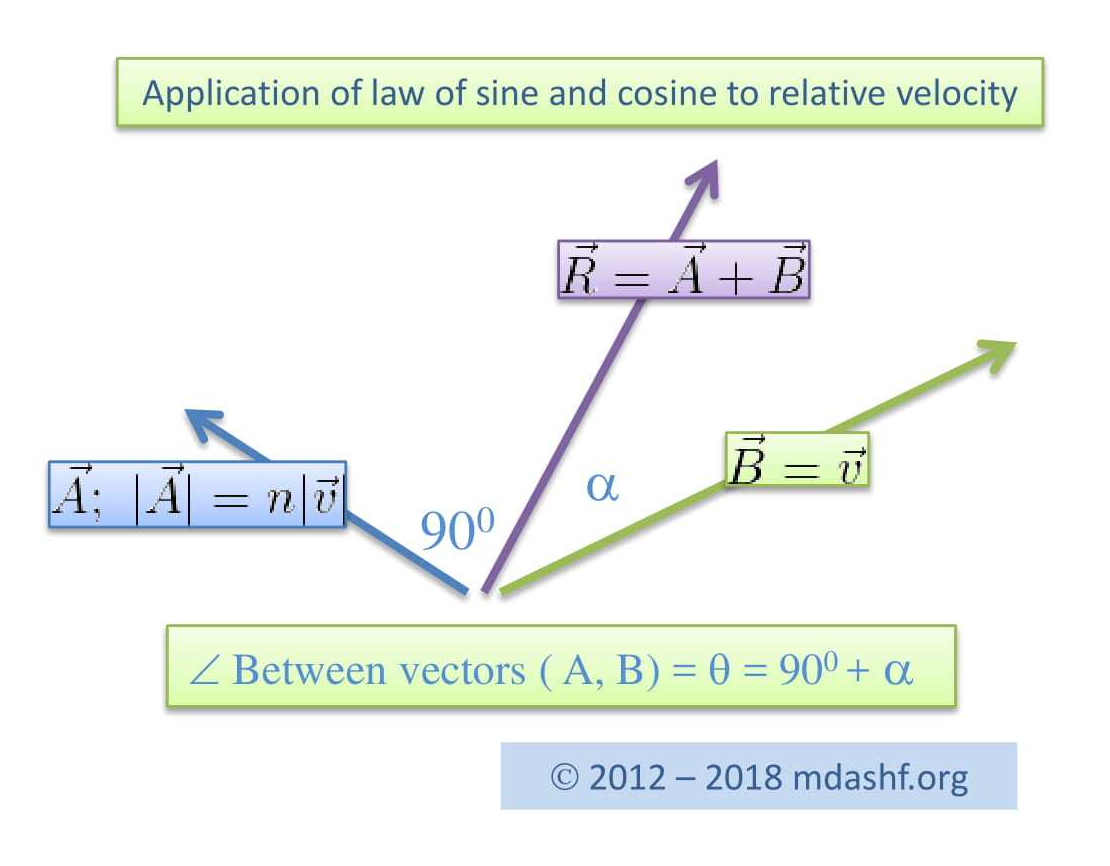
law of sine for relative velocity_edited

We instantly realize that “minimum drifting” is nothing but a direction which is $\perp$ to stream velocity or flow of water. That is our resultant direction should be such that there is zero impact along water flow.

Lets use law of sines with this in mind.

Law of sines; $\sin\alpha = \frac{B}{R}\sin\theta$ where $R$ is resultant boat motion. $\vec{R} = \vec{A} + \vec {B}$ , $(\vec{A}, \vec{B})$ make angle $\theta$ between them and $(\vec{R}, \vec{B})$ make angle $\alpha$ .

We realize that $\theta = \frac{\pi}{2} + \alpha$ is the angle made by $\vec{B} = \vec{v}$ = original velocity of boat, which is wrt frame associated with water-flow, with velocity of flow of water — wrt ground, given by $|\vec{A}| = n|\vec{v}|$ .

$\vec{R}$ : velocity of boat wrt ground-frame.

$|\vec{A}| = |\vec{v}|$ : velocity of water flow wrt ground.

Also $R$ is the — magnitude of, relative velocity of boat wrt ground, which is obtained from resultant of two velocity vectors, one of water wrt ground the other of boat wrt water. So its magnitude is given by law of cosines.

$R= \sqrt{A^2+B^2+2AB\cos(\frac{\pi}{2}+\alpha)}=\sqrt{v^2+(nv)^2+2v(nv) \cos(\frac{\pi}{2}+\alpha)}$ .

Use this into law of sines and the fact that $\sin(\frac{\pi}{2}+\alpha)=\sin\alpha, \,\, \cos(\frac{\pi}{2}+\alpha)=-\sin\alpha$ leads to;

$\sin\alpha = \frac{\sin(90+\alpha)}{\sqrt{1+n^2+2n\cos(90+\alpha)}}$ .

⇒ $1+n^2-2n\sin\alpha=1$

⇒ $n^2-2n\sin\alpha=1$ or $\sin\alpha = \frac{1}{n}$ .

Thus the boat must make an angle $\boxed{\frac{\pi}{2} + \arcsin\frac{1}{n}}$ with the stream direction.

Problem 9 — Irodov 1.10

Two bodies were thrown simultaneously from the same point: one straight up and the other at an angle of $\theta = 60^0$ to the horizontal. The initial velocity of each body is equal to $v_0 = 25 \,\frac {m}{s}$ .

Neglecting the air-drag, find the distance between the bodies, $t=1.7 s$ later.

Answer to Problem 9 — Irodov 1.10

Let us first describe the given problem in terms of a simple diagram.

projectile_edited

$S_x(A) = v_0 \sin{\theta} ^{'}t ,\, S_y(A) = (v_0 \cos \theta ^{'})t - \frac {1}{2} gt^2 ,\, S_y(B) = v_0 t - \frac{1}{2} gt^2$ .

$S = \sqrt{ [S_y(B)-S_y(A) ]^2+S_x(A)^2}=\sqrt{(v_0 \sin \theta ' t)^2+[v_0t(1-\cos\theta ')]^2}$ .

$\boxed{S =v_0 t\sqrt{\sin ^2 \theta '+1+\cos^2 \theta ' -2 \cos \theta '} = v_0 t \sqrt{2(1-\cos \theta ')} =v_0 t \sqrt{2(1-\sin \theta)} }$ .

Using given values the answer is: $22 \, m$

Problem 10 — Irodov 1.11

Two particle move in a uniform gravitational field with an acceleration $g$ . At the initial moment the particles were located at one point and moved with velocities $v_1 = 3.0 \frac{m}{s}, \,\,v_2 = 4.0 \frac{m}{s}$ horizontally in opposite directions.

Find the distance between the particles at the moment when their velocity vectors become mutually perpendicular.

Answer to problem 10 — Irodov 1.11

I have developed a concise method for this problem — after scribbling unsuccessfully for many pages.

Lets begin with a diagram.

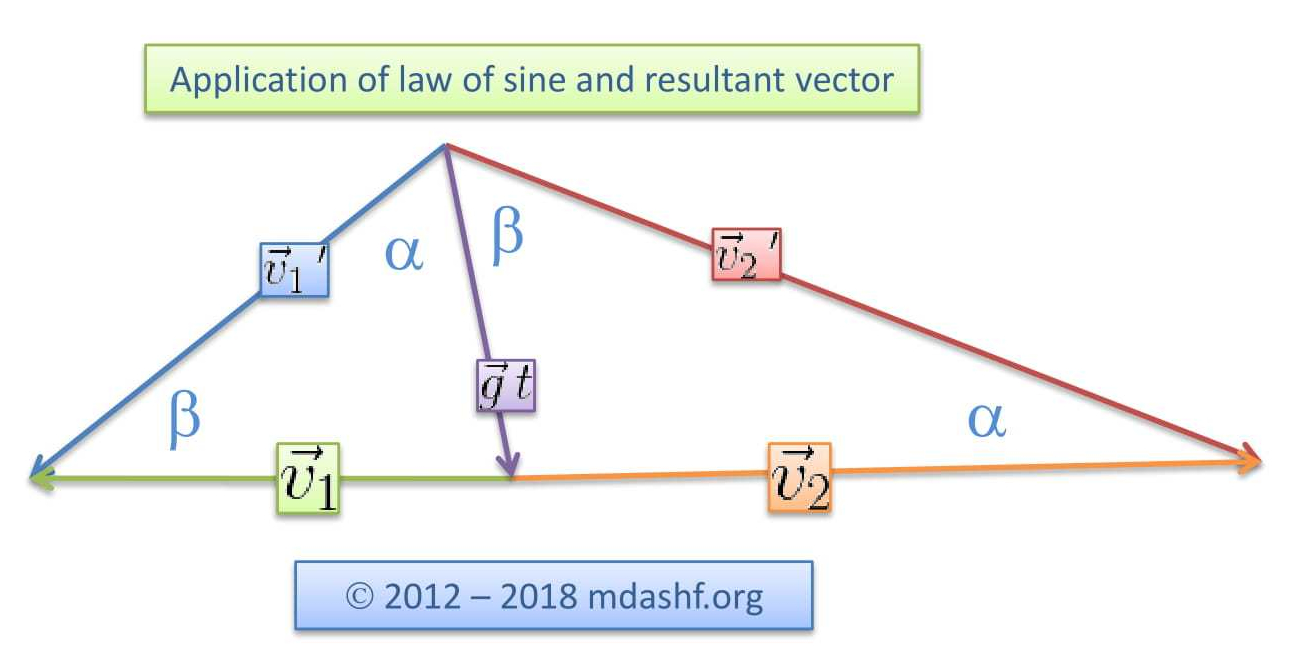
law of sines and resultant vector_edited

The velocities $\vec{v}_1, \,\vec{v}_2$ are horizontal. The resultant velocities $\vec{v}_1\,', \, \vec{v}_2\,'$ are because of the acceleration $\vec{g}$ generated by the field, in the vertical direction.

In a time $t$ . the resultant velocities become perpendicular to each other. i.e. $\alpha + \beta = \frac{\pi}{2}$ .

Let us apply “law of sines” to the congruent Δs — traingles.

$\frac{1}{v_2\,'}= \frac{\sin\alpha}{gt}=\frac{\sin\beta}{v_2}; \,\, \frac{\sin\alpha}{v_1}= \frac{\sin\beta}{gt}=\frac{1}{v_1\,'}$ .

⇒ $\frac{gt\sin\beta}{v_2} = \frac{v_1 \sin\beta}{gt}$ or $v_1 v_2 = g^2 t^2, \,\, t= \frac{\sqrt{v_1v_2}}{g}$ .

The distance between the particles are: $l = v_1 t + v_2 t = (v_1 + v_2) t = \frac{(v_1 + v_2) \sqrt{v_1 v_2}}{g}$ .

Using the given values: $l = 2.5 \,m$ .

Rate this:

Mahisapat, Dhenkanal, Odisha 759001, India

Comments

One response to “Solutions to Irodov problems”

Introduction to special theory of relativity. – "Invariance" from M Dash Foundation

October 21, 2018

[…] velocity addition and velocity transformation are the exact same thing. Read more about these here and here. The second link also expounds on what happens when speeds approach that of […]

LikeLike