Solid state physics, Lecture — III, IV
All articles in this series can be found here.
This article is purported to serve as an introduction to a solid state physics course for the 3 year degree physics honors class. It was delivered to the same class on 26th July 2017. Expect some refinement, add-ons, content expansion etc, in the web version.

Topics covered
a. Types and classes of crystals,
b. Symmetry operations in crystals
In this lecture we will follow through our basic knowledge gained in the last lecture, — lecture — I, II, and shed light on the most interesting properties of crystal lattices, viz. their symmetry properties. Based on their properties we will classify them into various types and classes.
i. In our last two lectures, here, — we saw that all lattices must satisfy a translational symmetry given by the lattice displacement vectors which qualifies them to be known as Bravais lattice.
ii. Lattices satisfy additional symmetry operations. But due to the constraint of translational symmetry the total number of symmetry operations that the lattices can satisfy is reduced to a minimum.
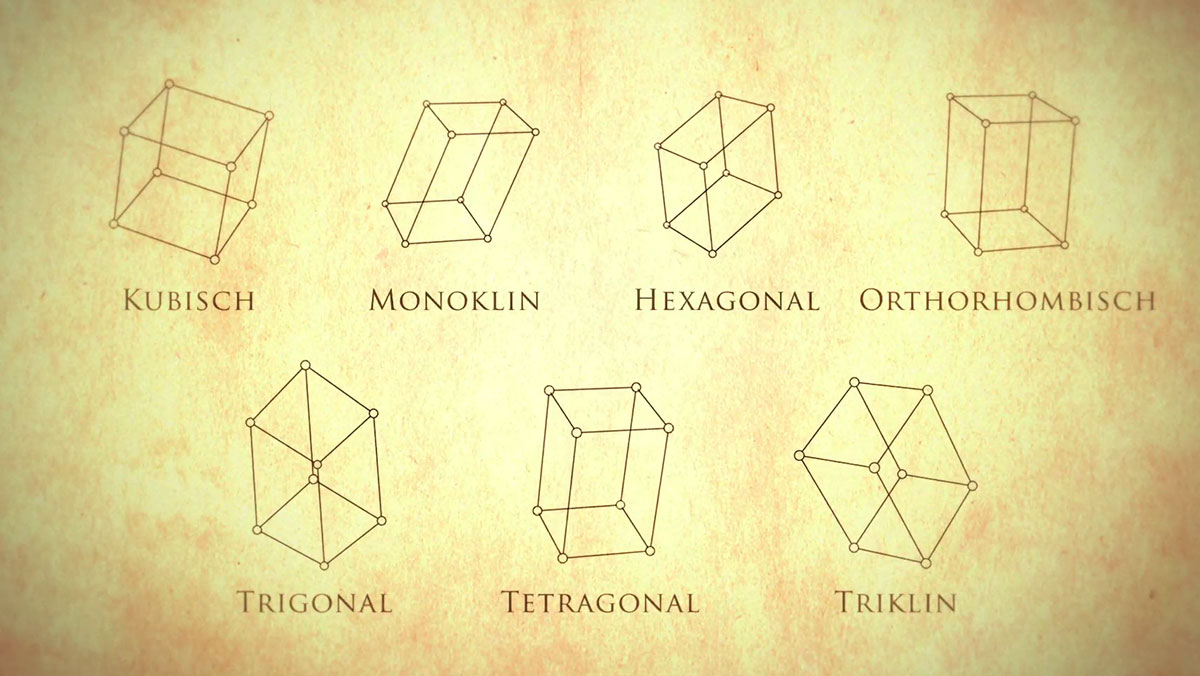
iii. This means in 2-dimensional lattice constructs we have only 5 types of lattices which satisfy additional symmetry operations. In 3-dimensional geometry there are a total of 14 classes of lattices.
iv. Thus in 3-dimensional lattices the 14 classes of Bravais lattices are categorized into 7 types or systems of fundamental lattices.
v. The extra symmetry operations are
- various rotations,
- inversion about a space point and
- reflection about a plane passing through a lattice point or
- their possible combinations.
vi. There are further symmetry operations that non-Bravais lattices such as “lattices with a basis” must satisfy. These symmetry are referred to as “point group symmetry” and together with obedience to translational symmetry referred as “space group symmetries“. There are only 230 such fundamental symmetry operations.
vii. A rotation is a transformation through angles of only, about an axis passing through a lattice point so that the lattice symmetry — translational that is, is preserved. The 5-fold symmetry is not possible and 1-fold symmetry is trivial.
viii. Inversion symmetry: A lattice point is taken as origin. All other lattice points given by are transformed to
so that lattice — translational symmetry, is preserved.
ix. Reflection symmetry: Its transformed through a plane about which a reflection or mirror image of all points retain their symmetry of the lattice.
When conditions are imposed on primitive lattice vectors — read lecture — I, II, linked here, to know how we define them, that is relations are established that restrict their size and the angle between the various dimensions, the resulting lattices are known as special lattice types, in 2-dimension there are 5 and in 3-dimension there are 14.
We show them below in the diagrams.
The first figure shows; oblique, square and hexagonal lattice.

The figure below shows; rectangular and centered rectangular lattices.

The following table shows the 14 types of special lattice types with their various properties.
| System | Type of lattice | Restriction on cell | Symmetry | Bravais lattice (B) | Point groups (P) | Product (BP) |
| Triclinic | Simple | Trivial | 1 | 2 | 2 | |
| Monoclinic |
|
One, 2-fold | 2 | 3 | 6 | |
| Orthorhombic |
|
Three mutually ⊥ 2-fold | 4 | 3 | 12 | |
| Tetragonal |
|
one, 4-fold | 2 | 7 | 14 | |
| Cubic |
|
Four, 3-fold | 3 | 5 | 15 | |
| Trigonal or Rhombohedral | Simple | One, 3-fold | 1 | 5 | 5 | |
| Hexagonal | Simple | One, 3-fold | 1 | 7 | 7 |
Now in the following table we will summarize the properties of the 3 most important lattice types, the simple cubic (sc), body centered cubic (bcc) and face centered cubic (fcc) types.
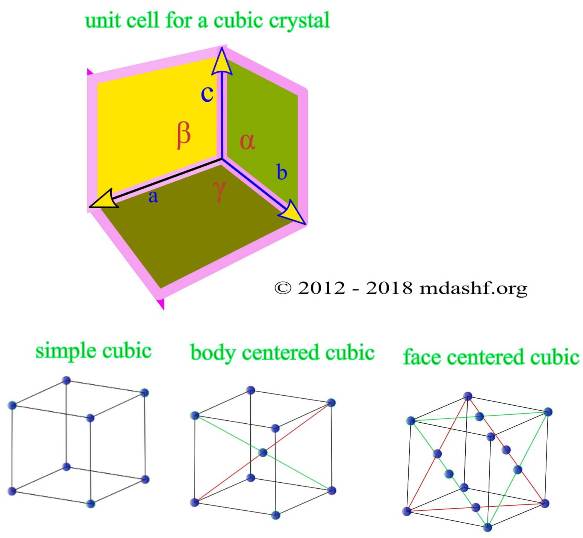
| Parameter | simple cubic | body centered cubic | face centered cubic |
| Volume of conventional cell | |||
| Lattice points per cell | |||
| Nearest neighbors | |||
| Volume of primitive cell | |||
| Lattice points per unit cell | |||
| Nearest neighbor distance | |||
| Second neighbors | |||
| Distance to second neighbor | |||
| Packing fraction |
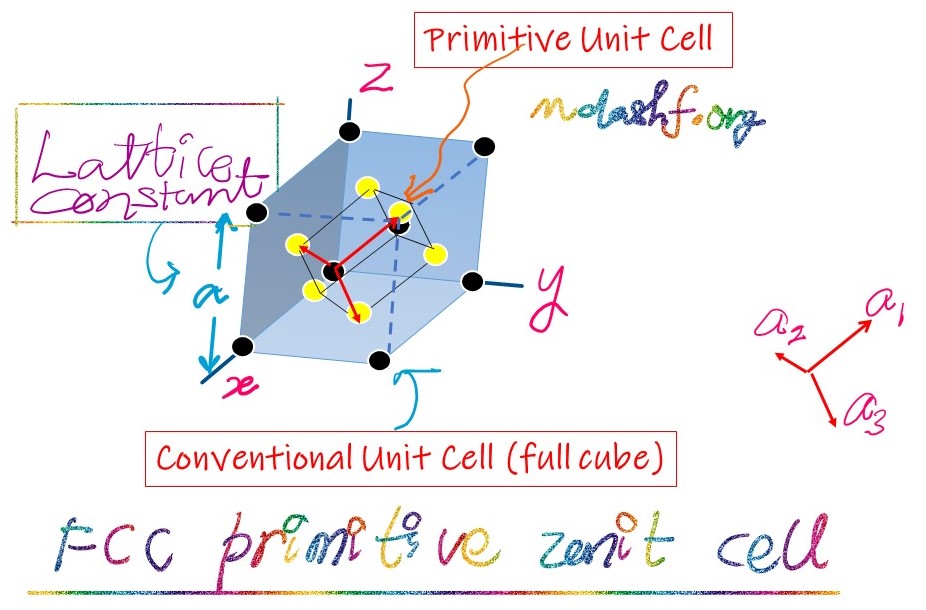
Primitive cells of cubic crystals
Home-work: you should try to understand the primitive cells of other types of crystals.

Body centered cubic: Lattice point at origin is connected to lattice point at body centers of 3 cubes whose intersection is origin. Primitive cell is a rhombohedron. This is a primitive unit cell different from the conventional cell. See images below.


Face centered cubic: Lattice point at origin — at corner, is connected to lattice point at face. Primitive cell is a rhombohedron.
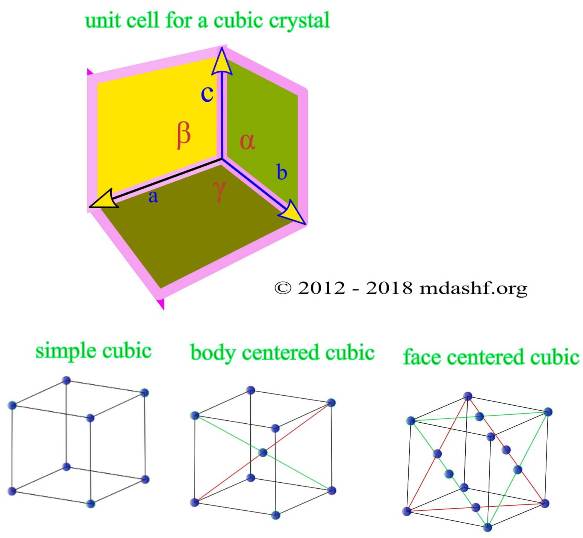
Leave a comment